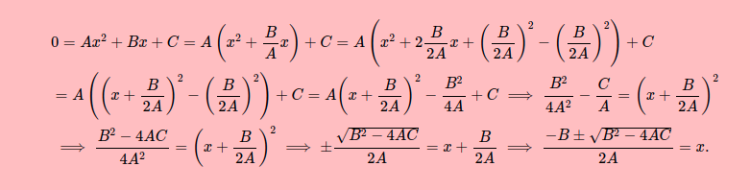APPM Homework Style Guide

• Use enough space
• Problems should be labeled and in order.
• Have at most two columns
• Separate problems vertically

Use Enough Space: Be sure you have 1-inch margins. If you are using a two column layout, be sure the inner margins are large enough (see examples below). You should afford yourself as much space as you need for each problem. You should label each problem, and you should organize the problems on each page in a clear and consistent way. If a problem spans multiple pages, you should label the pages in a way that makes their order clear.

Labeled in Order: Clearly label each problem, and write solutions in order. If problems span multiple pages, label each problem on each page, and make it clear it is a continuation from a previous page. If using a two column format, problems should be presented sequentially from top to bottom within the first column, then top to bottom within the second column.

At Most Two Columns: Do not use more than two columns. Use either the entire page (one column) or split the page down the middle (two columns). If you are using two columns, be sure to follow the margin guidelines and to leave yourself enough space.

Vertical Separation: You should be able to draw horizontal lines that clearly separate each problem (within a column).

We recommend that you have at most one problem on each page. This is the easiest way to ensure that you have enough space if you complete the problems in an order different from the order in which they are presented.

Example 1: Good Layouts

Single Column: Note that problems are separated vertically, and clearly labeled.

 

Two Columns: Note that problems are separated vertically within each column, clearly labeled, and in order.

Example 2: Bad Layout

Note that problems 1 and 2 cannot be separated by a straight horizontal line, and similarly for problems 2 and 3. This layout is confusing. The author recognized this, and tried to help explain the progression of steps using arrows. Do not do this. Also note that this is not clearly labeled. The sections for problems 2 and 3 have two upper-left corners. The label for problem 2 is in the uppermost upper-left corner and the label for problem 3 is in the leftmost upper-left corner. This is inconsistent and confusing. When problems are vertically separated it becomes easy to label problems clearly.

Your submitted homework should be polished and clear. Generally, when we work through a math problem we may make mistakes and erase or strike-through incorrect steps or sub-expressions. This is natural, as written mathematics is a tool we use to reason through a problem in addition to a means of communicating ideas. However, your submitted work should follow a higher standard.

In particular, your submitted homework should:

• be clear and legible, with no eraser marks (a few are alright as long as they don't inhibit readability) and no crossed-out work
• have good scan quality with pages associated to problems (when submitting online)
• be stapled (when submitting in person) with the pages in order
• have clean pages, no crumples or folds, remove the "notebook frills"

It is generally a good idea to work a problem out on a whiteboard or some scratch paper, and compare with your study group before neatly transcribing it onto your homework.

If you are using new symbols or characters from an unfamiliar alphabet, take the time to practice your penmanship. In particular, make sure that the character/symbol is easily recognized and easily differentiated from other characters/symbols in your handwriting.

Example 1: Clear and Legible

The following example is clear and legible. You will find some eraser marks if you look carefully, but they are not noticeable and do not inhibit readability.

Example 2: Eraser Marks and Crossed Out Work

The following example has crossed out areas, and significant eraser marks that inhibit readability. Do your problems on scratch work and neatly transcribe them to your homework to avoid this.

Example 3: Scan Quality

Even if your homework is very clear and legible, a poor scan can render it unreadable. If you are struggling, try changing the lighting, or background, or try a different scanning application. Be sure your scan does not cut off portions of the page.

When manipulating expressions, always communicate how they relate to each other. In most cases, this is as simple as using the equals sign: "=". Do not omit the equals sign when you mean to communicate that two expressions are equal. Do not use other symbols (such as arrows) when you mean to communicate that two expressions are equal. If two steps relate through something other than equality then explain this relationship, using words if necessary.

In summary:

• Explain how your expressions relate.
• Use equal signs when appropriate.
• Do not omit equal signs when you mean to communicate that two expressions are equal.
• Do not use other symbols in place of equals signs.
• Use words if necessary.

Example 1: Good

The following example derives the quadratic formula clearly and correctly. Notice that equals signs are used between expressions that are equal, and text is used to separate the work when we begin to manipulate a sub-expression, and when we substitute.

Example 2: Bad - Omits Equals

Do not omit the equals sign assuming that it is obvious how your expressions relate. The following example fails to do this. A reader might infer that these expressions relate to each other through equality. It becomes particularly difficult to understand when they complete the square and the expressions are unequal.

Example 3: Bad - Uses Incorrect Symbols

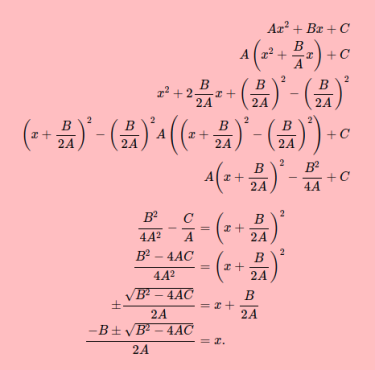
Do not use other symbols, when you mean to communicate that two expressions are equal. The following examples attempt to use other symbols instead. Some of these have precise mathematical meanings and are syntactically incorrect.

Long symbolic manipulations should be stacked vertically, opposed to in-line. Vertically separated expressions are much easier to read and validate. Reader comprehension is more important than space-efficiency. You may split your page into columns, however, be sure that you maintain other style requirements (such as 1-inch margins) and that it is clear how to read your work.

Example 1: Good - Vertical Math

The following example displays a sequence of manipulations vertically. It is easy to tell which expressions are equivalent, and what steps were taken.

Example 2: Good - Parallel Work in Separate Columns

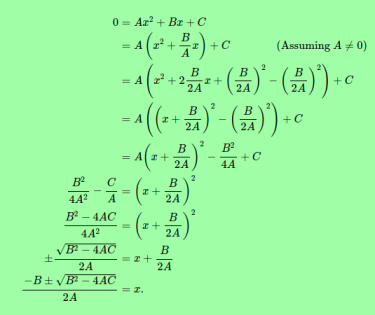
The following demonstrates an acceptable use of multiple columns. Note that they explain that it is clear that the order does not matter (independent integrals) and that they maintain margin spacing.

Example 3: Bad - Horizontal Math

Do not submit homework with long algebraic manipulations done horizontally: